Vibration G-force Definition and Calculation
So called G-force, it is actually not a force. G-force is an acceleration expressed using G as the unit. G is referred to as the acceleration of gravity, which equals 9.81m/s2 at the sea level.
Acceleration of an object is defined as the rate of its velocity change (Δv) in a given period of time (Δt).
Acceleration a = Δv / Δt
Acceleration is commonly expressed using the unit m/s2. When it is related to acceleration's influence to human body, G-force is usually adopted to express acceleration so that its magnitude can be convenient comprehended, because on earth we experience the acceleration of gravity(G) all day long.
G-force is calculated as acceleration in m/s2 divided by G (9.81 m/s2). For a precise definition, G-force is an acceleration ratio that is related to the acceleration of gravity. For example, that a rocket moves up on a 2G acceleration during the launch means its acceleration is 2 times of the acceleration of gravity.
For a vibration plate, G-force is an important indicator of vibration intensity. The higher the G-force, the higher the influence the vibration plate can put on human body.
Non-Constant Acceleration

Vibration motion is in repeated cycles. In each cycle the velocity changes from zero to maximum, maximum to zero and repeats in reverse direction: v = ωDcos(ωt). The G-force of vibration keeps changing its value between the max positive value and the max negative value in each vibration cycle.
In physics, simple harmonic vibration model is used to analyze and calculate vibration acceleration. With this model, velocity and time are replaced by frequency and amplitude in the calculation.
The acceleration formula of simple harmonic vibration model is set as below.
a = -(2πf)2 Asin(2πft)
a: acceleration in m/s2
π: 3.14
f: vibration frequency in Hz
A: vibration amplitude in meter
t: time in second
The factor sin(2πft) has its value changing from -1 to 1 on the time line of a vibration cycle. It depicts that the acceleration changes from the max negative positive value to the max positive value.
It is important to note that the amplitude in this formula is the amplitude defined in physics. The "amplitude" quoted by all vibration plate manufacturers is actually peak-to-peak amplitude, which doubles the amplitude defined in physics.
Therefore, using this formula, the "amplitude" quoted by manufacturers should be cut by half in order to correctly calculate G-force. Otherwise, the G-force value result is incorrectly doubled.
Nominal Vibration Acceleration
Vibration acceleration is not a constant value. However, for a vibration plate, we want to use a nominal value to represent the vibration acceleration, so that we can properly reference, evaluate and compare the intensity of different vibration plates.
The nominal acceleration should also allow us to properly evaluate the influence of acceleration on our body, comparable to the influence of other typical types of acceleration, like acceleration from riding a rocket, an airplane or an elevator.
How do we find a nominal acceleration?
For a vertical vibration plate, its non-constant vibration acceleration influences us differently in each of 4 phases of a vibration cycle. Let's look at how the platform of a vibration plate moves and influences us in these four phases.
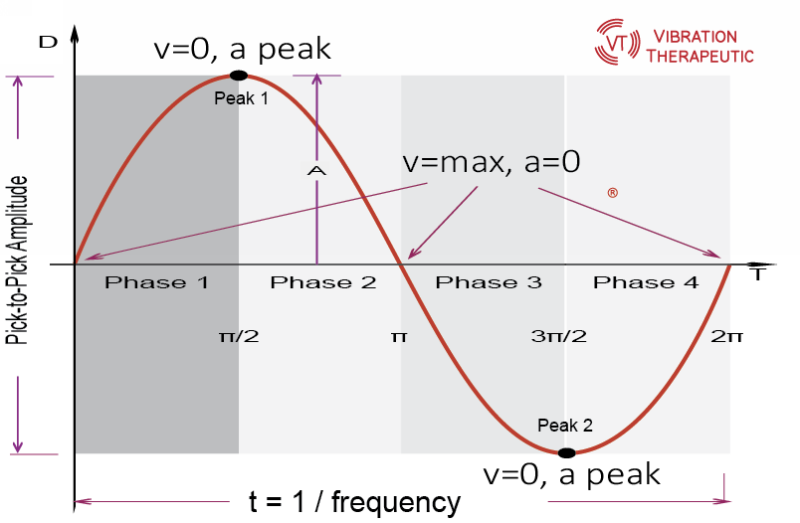
4 Phases of a Simple Harmonic Vibration
| Phase 1 | The platform travels from equilibrium point to the highest point (peak) in a decelerated velocity. The acceleration changes from 0 to the max negative value. When the vibration platform decelerates faster than the acceleration of gravity, user's body may not stick on the platform. The body can be thrown up on a projectile motion. In Phase 1, the vibration plate form does not push the users. The acceleration's influence to our body can be ignored. |
| Phase 2 | The platform changes its movement direction at the peak point and travel back to equilibrium point. The acceleration changes from max negative to 0. The platform moves down in accelerated velocity, at the point the acceleration rate is greater than G, user's body is in free-fall motion. In Phase 2, vibration platform does not push the users. The acceleration's influence to our body can be ignored. |
| Phase 3 | The platform continues to travel to the lowest point (the other peak) ?in decelerating velocity. The acceleration changes from 0 to the max positive value. The user's body continues with free fall and then catch up with the platform when the acceleration is less than G. In Phase 3, the vibration platform does not push the users. The acceleration's influence to our body can be ignored. |
| Phase 4 | The platform changes direction at the lowest point and travels back to the equilibrium point. The acceleration changes from max positive to 0. This finishes a whole vibration cycle. In Phase 4, the vibration platform pushes up the users. This is the only phase that the vibration platform actually apply a pushing force on user's body. |
Phase 4 Vibration Acceleration
In a vibration cycle, phase 4 is the only phase that the vertical vibration platform actually applies a pushing force on user's body. The acceleration in phase 4 represents the intensity of the vibration plate.
In Phase 4, the vibration platform moves up in a similar manner of a launching rocket, which is typical. During launch, the rocket starts with the highest upward acceleration. Then the acceleration gradually reduces to zero as the rocket speed gradually increases and reaches plateau. This is the same when the vibration platform moves from the lowest point to the equilibrium point. It is just that rocket launch lasts a few minutes; while the Phase 4 vibration movement lasts only a tiny fraction of a second (1/4 of vibration cycle).
Phase 4 acceleration properly represents vibration intensity and the impact to human body. It is comparable with other typical types of acceleration in terms of the influence to human body. It fits the best as the nominal vibration acceleration of a vibration plate.
Within Phase 4, the acceleration is not mixed with positive and negative values. It is easy to find the rate of velocity change (Δv) of the entire phase in the time period of the phase (Δt).
The simple harmonic vibration model has provided the velocity formula:
v = (2πf)Acos(2πft)
The factor cos(2πft) changes from 0 to 1 in the time line of Phase 4. It expresses that, as the vibration platform travels from the lowest point to the equilibrium point, the velocity changes from 0 to (2πf)A.
Δv = (2πf)A
Phase 4 is 1/4 vibration cycle, so the time period is
Δt = 1/4(1/f) = 1/(4f)
Therefore,
Phase 4 acceleration = Δv/Δt = 8πf2 A = 25.12f2A
This is the formula of nominal vibration acceleration of vibration plate.
Nominal Vibration G-force
Let's convert the Phase 4 acceleration to Phase 4 G-force.
Phase 4 G-force = 25.12f2A/9.81 = 2.56f2A
f: vibration frequency in Hz
A: vibration amplitude in meter
Replace the unit of amplitude from meter to mini meter:
Phase 4 G-force = 0.00256f2A
Phase 4 G-force is the nominal vibration G-force that best represents the G-force of a vibration plate in terms of vibration intensity and the influence on human body. It is also comparable to other typical forms of acceleration, like from riding a rocket, an airplane, or an elevator.
Now we have the nominal vibration G-force formula for a vibration plate.
Vibration G-force = 0.00256f2A
f: vibration frequency in Hz
A: vibration amplitude in mm
Note: the amplitude in this formula is the amplitude defined in physics. The amplitude quoted by all vibration plate manufacturers is actually peak-to-peak amplitude, which is 2 times of the amplitude defined in physics. Therefore, using this formula, the "amplitude" quoted by manufacturers should be cut by half in order to correctly calculate G-force. Otherwise, the G-force value is incorrectly doubled.
To reiterate, the G-force of vibration is a non-constant value. We take the Phase 4 G-force as a nominal vibration G-force to reference, compare and assess vibration intensity for studying its influence to human body.
Vibration G-force's Impact on Human Body
On a vibration plate, vibration acceleration creates additional force on human body. It is calculated as the body weight multiplied by the G-force.
For example, you stand on a vibration plate running on 2G acceleration. You weigh 200Lb. In the 4th phase of a vibration cycle, you will experience a push-up force of 2x200LB=400Lb. You would feel a pull-down counter force of 400Lb. Adding your own body weight, he would feel as if your body weigh 400+200=600Lb. However, you may not perceive such a heavy weight, because pulling-down force only lasts a tiny fraction of a second (1/4 cycle) in each vibration cycle. If the vibration plate runs on 30Hz, this tiny period of time is 0.008 second.
Influence of Acceleration of Gravity
On earth, our body is subject to the acceleration of gravity (1G) all the time, which translate to the body weight we feel.
When on a running vibration plate, our body is subject to a combined G-force of the natural acceleration of gravity (1G) and the vibration G-force.
On the vertical direction, the combined G-force is calculated by simply adding 1G and the vibration G-force, because both accelerations are on the same vertical direction.
On the horizontal direction, the combined G-force is calculated by combining two vectors using Pythagorean theorem.
For vibration plates, we only focus on vertical direction G-force which provides the right pushing force that can be used as a treatment intervention.
Vibration G-Force Calculation Examples
G-Force of VT003F Vibration Plate

For Model VT003F vibration plate , the max G-force occurs at the frequency 40Hz, and peak-to-peak amplitude 1.5mm (A=0.75mm).
The nominal vibration G-force is calculated as below:
G-Force of VT007 Vibration Plate

For Model VT007 vibration plate , the max G-force occurs at the frequency 40Hz, and peak-to-peak amplitude 1.6mm (A=0.8mm).
The nominal vibration G-force is calculated as below:
G-force of VT027 Pivotal Oscillation Plate

For Model VT027 vibration plate , given the highest frequency 14Hz, and the highest peak-to-peak amplitude is 10mm (A=5mm).
The nominal vibration G-force is calculated as below:
Body Weight and G-force
Body weight is not a variable in G-force's calculation. However, for linear vibration plate, the amplitude yield to the user's body weight. So different body weight causes different G-force.
For pivotal oscillation type of vibration plate, body weight does not affect G-force.